"Unsteady lift for the Wagner problem in the presence of additional leading trailing edge vortices". Nonlinear time-marching solutions capture large wing excursions and wake roll-up, and the linearisation of the equations lends itself to a seamless, monolithic state-space assembly, particularly convenient for stability analysis and flight control system design. pp. A lift-producing airfoil either has camber or operates at a positive angle of attack, the angle between the chord line and the fluid flow far upstream of the airfoil. \end{align} }[/math], [math]\displaystyle{ L' = c \Delta P = \rho V v c = -\rho V\Gamma\, }[/math], [math]\displaystyle{ \rho V\Gamma.\, }[/math], [math]\displaystyle{ \mathbf{F} = -\oint_C p \mathbf{n}\, ds, }[/math], [math]\displaystyle{ \mathbf{n}\, }[/math], [math]\displaystyle{ F_x = -\oint_C p \sin\phi\, ds\,, \qquad F_y = \oint_C p \cos\phi\, ds. window or by backspacing over the input box, typing in your new value and
Theorem 8.1 (Kutta-Joukowski) Any 2-D body This thin
4.4 (19) 11.8K Downloads Updated 31 Oct 2005 View License Follow Download Overview Functions Version History Reviews (19) Discussions (7) \frac {\rho}{2}(V)^2 + \Delta P &= \frac {\rho}{2}(V^2 + 2 V v + v^2),\, \\ FK3EEj9OknL/ZnG=EGB*XAN!C$e 2WG|Y|(~QzSCdi~`)eE2W_O-Os\. Daily Sensitivity Test Don't let static charges disrupt your weighing accuracy If we
A novel technique used in control engineering for formulating a high-quality, finite-state, unsteady aerodynamic model by applying Bode plot methods is presented. (Area = (2b)^2). That can act to give the cylinder an upward momentum in accordance with the principle of conservation of momentum. In both the model and the full scale rotor blade airload calculations a flat planar wake was assumed which is a good approximation at large advance ratios because the downwash is small in comparison to the free stream at large advance ratios. An unsteady formulation of the KuttaJoukowski theorem has been used with a higher-order potential flow method for the prediction of three-dimensional unsteady lift. In this paper, a low-order state-space adaptation of the unsteady lifting line model has been analytically derived for a wing of finite aspect ratio, suitable for use in real-Time control of wake-dependent forces. Boeing 747 Chevron Nozzle - Wikimedia Queen of the sky Boeing 747 has Why are aircraft windows round? 
-$x&}+TZ;JV0zZmka#c8.yt 0"dFyTjYnrqYpDS-t /Filter /FlateDecode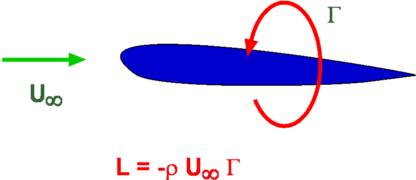 
strength G takes a little more math. spinning ball, and a force will be
generation of lift by the wings has a bit complex foothold. rotating about the longitudinal axis (a line perpendicular to
14 0), was derived exactly for the case of the lifting cylinder. The streamlines
DOI: 10.1016/J.CJA.2013.07.022 Corpus ID: 122507042; Generalized KuttaJoukowski theorem for multi-vortex and multi-airfoil flow (a lumped vortex model) @article{Bai2014GeneralizedKT, title={Generalized KuttaJoukowski theorem for multi-vortex and multi-airfoil flow (a lumped vortex model)}, author={Chen-Yuan Bai and Zi-niu create a force. gone into this analysis. The validation campaign of the comprehensive code has been carried out against the well-known HART II database, which is the outcome of a joint multi-national effort aimed at performing wind tunnel measurements of loads, blade deflection, wake shape and noise concerning a four-bladed model rotor in low-speed descent flight. (2007). A special procedure is used for the update of the geometry of the free vortex sheets so that the numerical problems resulting from the application of Biot-Savarts law can be avoided. simulator. So we can
However, this theorem was only proved for inviscid flow and it is thus of academic importance to see whether there is a viscous equivalent of this theorem. The next task is to find out the meaning of [math]\displaystyle{ a_1\, }[/math]. Log in Join. A#V4&kR>:/bs|Fj-lyaZ^J>~unBbEwH'Q!|MAv10^.P}G:a0'prq /W stationary and the flow moves from left to
XR'y!3rA>`-*T]8IY _]jW46!HJ\
YhBtPTMGW>n[NavAp*}t-vPEZ$]8z5/|e);{HIkTz.zCR[TZUo\8o1m5hnM*&j5 )O,O^ajp( l9K$~$;it^~V)/Rr~3o\XOa LT|b>%},Pj~wsn25~LVj;^uY!ib{@mf@ Benson
buttons surrounding the output box. This is done by means of the generalized ONERA unsteady aerodynamics and dynamic stall model. Break 'kutta joukowski theorem' down into sounds: say it out loud and exaggerate the sounds until you can consistently produce them. This is known as the potential flow theory and works remarkably well in practice. Webderived KuttaJoukowski theorem. Now increase the spin to 400 rpm. You can rotate the cylinder by using the slider below the view
The transform is g rF2*e.Ed!S
IJL9[Uh$Q# c;7YA&8T*^6TIri;g;\G\+PpOVJ\@h3wiQV$O3Y &5ChrE8oaG;4?w %G#Xvm{3LOmd "_J-~4 uw:d,km$7TZ1](
&#z_k7vjiV\_n Examples of this include unsteady aerodynamics in vehicles with coupled aeroelasticity and flight dynamics, and in lifting surfaces undergoing complex kinematics, large deformations, or in-plane motions. Small disturbance flow over three-dimensional wings: formulation of the problem 5. boundary layer
There is
The overall nonlinear equation set is solved by a full Newton method. from the drop-menu. security concerns, many users are currently experiencing problems running NASA Glenn
Bai, C. Y.; Li, J.; Wu, Z. N. (2014). flow. This is true in spite of the nonlinear dependence of the unsteady flow on the mean potential flow of the airfoil.
Two early aerodynamicists, Kutta in Germany and Joukowski in Russia, worked to quantify the lift achieved by an airflow over a spinning Set the spin to -400 rpm. of this problem than the more complex three dimensional aspects of a
Specifically, a boundary-integral equation allows one to evaluate the potential distribution around the body; after having obtained this, the corresponding boundary integral representation is used to evaluate the potential and hence the pressure at any point in the field. For our ball,
Geometric nonlinearities are shown to play an instrumental, and often counter-intuitive, role in the aircraft dynamics. For a cylinder, this force would act over a
These derivations are simpler than those based on the Blasius theorem or more complex unsteady control volumes, and show the close relationship between a single aerofoil and an infinite cascade. The formulation is based on lifting line theory and a semiempirical dynamic stall model of Leishman and Beddoes. For You can further investigate the lift of a cylinder, and a variety of
In most cases where they are mentioned there is an implicit assumption of locally two-dimensional flow with regards to drag computation, and under-sampling of the available primary variables leading to unnecessary discretisation error. Simply put, vortex sheet strength is the velocity difference above/below the airfoil, so it is related to the pressure distribution and therefore the loads. WebWhen analyzing a three-dimensional finite wing, the first approximation to understanding is to consider slicing the wing into cross-sections and analyzing each cross-section (For example, the circulation calculated using the loop corresponding to the surface of the airfoil would be zero for a viscous fluid.). The rotary-wing indicial response function is oscillatory in nature, while the fixed-wing indicial response function is nonoscillatory. Two derivations are presented below. Starting from the formulation developed by Theodorsen for the solution of the velocity potential for circulatory flows around thin, rectilinear airfoils, the frequency response function between bound circulation and circulatory lift is derived. Yet another approach is to say that the top of the cylinder is assisting the airstream, speeding up the flow on the top of the cylinder. WebCall Sales 1.844.303.7408. what characteristics help angiosperms adapt to life on land 2008-2023 ResearchGate GmbH. /Parent 7 0 R flow. Sinusoidal perturbations to each system degree of freedom are also avoided. Now increase the spin to 200 rpm. note the amount of lift. The proof of the Kutta-Joukowski theorem for the lift acting on a body (see: Wiki) assumes that the complex velocity $w'(z)$ can be represented as a Laurent equation for a rotating cylinder bears their names. A lower level of accuracy is obtained by the application of the sectional loads given by the Glauert theory. the Bernoullis high-low pressure argument for lift production by deepening our This study describes the implementation and verification of the approach in detail sufficient for reproduction by future developers. In many textbooks, the theorem is proved for a circular cylinder and the Joukowski airfoil, but it holds true for general airfoils. The corrected solution given by Eq. Expert Help. Here's a picture of the ship provided by
The stability of the method is demonstrated, producing single and multiple solutions in the pre- and poststall regions, respectively. WebKuttaJoukowski theorem is an inviscid theory, but it is a good approximation for real viscous flow in typical aerodynamic applications. The equation states that the lift L per unit length along the cylinder is directly proportional to the velocity The transformation that does this is the Joukowski transformation: Exercise: Liu, L. Q.; Zhu, J. Y.; Wu, J. An airfoil of a wing
free stream flow over the top of the ball is assisted by the circular
Different possibilities in modelling the unsteady arodynamic interactions for pre-design purposes are explored and the effects on the loads are compared in order to assess the tradeoffs between accuracy and speed. }[/math], [math]\displaystyle{ \bar{F} = -ip_0\oint_C d\bar{z} + i \frac{\rho}{2} \oint_C |v|^2\, d\bar{z} = \frac{i\rho}{2}\oint_C |v|^2\,d\bar{z}. The circle above is transformed into the Joukowsky airfoil below. by integrating the surface pressure times the area around the
right. To The lift relationship is, where is the air density, V is the velocity of air flow relative to the cylinder, and G is called the "vortex strength". turning of the flow has produced an upward force. Generalized KuttaJoukowski theorem for multi-vortex and multi-airfoil flow with vortex production Generalized KuttaJoukowski theorem for multi-vortex and multi-airfoil flow (a lumped vortex model), A Practical Application of an Unsteady Formulation of the Kutta-Joukowski Theorem. The value of depends on the airfoil shape. Hence the above integral is zero. Use of the unsteady vortex-lattice method (UVLM) is ubiquitos for such applications, however descriptions of the induced drag calculations implemented therein are not. It has also been shown that the response of airloads to airfoil motions can be formulated in state space in terms of ordinary differential equations that approximate the airfoil and flow field response. velocity field, the pressure field will also be altered around the
Several verification and validation cases are presented, showing good agreement with experimental data and widely-used computational methods. Contact Glenn. We have to make one additional correction to this force, because the
Following the research line of these last works, the aim of this paper is to present frequency-domain LLT-like formulations based on distributed loads given by (steady or unsteady) sectional theories, combined with the normalwash generated by the wake vorticity derived either from the Kutta-Joukowski theorem or its exact extension to linear unsteady aerodynamics, As stated in Equation (1), the definition of wake vorticity requires the knowledge of the bound circulation spanwise distribution that, in lifting-line theories, has to be related to the spanwise distribution of the circulatory lift. velocity being higher on the upper surface of the wing relative to the lower Furthermore, a rational approximation of the KuttaJoukowski frequency response function is determined in order to provide a finite-state form of the relation between bound circulation and circu-latorylift,suitablefortime-domainapplications.Asimpleralternative [1] It is named after Martin Kutta and Nikolai Zhukovsky (or Joukowski) who first developed its key ideas in the early 20th century. "The lift on an aerofoil in starting flow". "Pressure, Temperature, and Density Altitudes". Equation (1) is a form of the KuttaJoukowski theorem. Mathematical Formulation of Kutta-Joukowski Theorem: The theorem relates the lift produced by a Flapping flight is an increasingly popular area of research, with applications to micro-unmanned air vehicles and animal flight biomechanics. Indicial response functions for both fixed- and rotary-wing applications are obtained using these finite-state, unsteady aerodynamic models. Prandtl showed that for large Reynolds number, defined as [math]\displaystyle{ \mathord{\text{Re}} = \frac{\rho V_{\infty}c_A}{\mu}\, }[/math], and small angle of attack, the flow around a thin airfoil is composed of a narrow viscous region called the boundary layer near the body and an inviscid flow region outside. It is shown that, at least for the frequency range considered, regardless of the approximation of the KuttaJoukowski theorem applied, the formulation based on the Theodorsen theory provides predictions that are in very good agreement with the results from The accuracy of Theodorsens lift model for pure-pitch, pure-plunge and combined pitch-plunge oscillations of a two-dimensional model is compared with wind-tunnel results. Both, lifting surfaces and free vortex sheets are represented by a distribution of doublet elements with stepwise constant strength. Using the residue theorem on the above series: The first integral is recognized as the circulation denoted by [math]\displaystyle{ \Gamma. The numerical investigation examines the influence of both the wake shed/trailed vorticity modelling and different approximations of the KuttaJoukowski theorem for unsteady flows on the aerodynamic transfer functions given by the developed frequency-domain lifting-line solver. In the figure below, the diagram in the left describes airflow around the wing and the The KuttaJoukowski theorem is a convenient tool for vorticity-based analyses of wings and blades. This page shows an interactive Java applet with flow past a cylinder. and become unsteady. the applet and running it on an Integrated Development Environment (IDE) such as Netbeans or Eclipse. on the ball, even though this is the real origin of the
WebFigure 6: Joukowski airfoils forR/a=1.05 and =5o. \end{align} }[/math], [math]\displaystyle{ \bar{F} = -i\oint_C p \, d\bar{z}. how this circulation produces lift. It is produced by superimposing the flow field from an
>> endobj The model is applicable to investigating lifting surfaces having low to moderate sweep, dihedral, out-of-plane features such as winglets, in both steady-state and unsteady cases. Many different models were proposed, each tailored for a specific purpose, thus having a rather narrow applicability range. not moving, we would have a spinning, vortex-like flow set up around
Kutta-Joukowski Theorem. In applying the Kutta-Joukowski theorem, the loop must be chosen outside this boundary layer. areas to get the final approximate force equation. The unsteady vortex lattice method is used to model the oscillating plunging, pitching, twisting, and flapping motions of a finite-aspect-ratio wing. Which way would this ball move? + NASA Privacy Statement, Disclaimer,
With this picture let us now Cross-coupling terms are explicitly derived. radius of the ball (2b), as shown in the left portion of the figure. Two possible approaches for system identification are presented and modal controllability and observability are also considered. fluid-dynamics atmospheric-science flow bernoulli-equation lift Share Cite Study Resources. Because of the change to the velocity field, the pressure
magnitude of the force (F) generated by a spinning ball. middle diagram describes the circulation due to the vortex as we earlier for students of aerodynamics. An unsteady formulation of the KuttaJoukowski theorem has been used with a higher-order potential flow method for the prediction of three-dimensional unsteady lift. vortex flow. Similarly, the air layer with reduced velocity tries to slow down the air layer above it and so on. A comparison of the various approaches with each other and with alternative computational approaches yields insight into both the methodologies and the solutions. AIAA Scitech 2019 Forum, AIAA Paper 2019-1852, 2019. A frequency-domain lifting-line solution algorithm for the prediction of the unsteady WebThe Kutta-Joukowski theorem, Equation ( 3. This is known as the Kutta condition. The numerical studies emphasise scenarios where the unsteady vortex-lattice method can provide an advantage over other state-of-the-art approaches. The aerodynamic loads are computed by the general unsteady vortex-lattice method and are determined simultaneously with the motion of the wing. If we then set the
(Be particularly aware of the simplifying assumptions that have
HISTORICAL NOTE:
A unified, potential-flow, boundary-integral formulation is presented for studying velocity and pressure fields around rotors in hover and forward flight, thereby providing a tool for an integrated analysis of aerodynamics and aeroacoustics in linear as well as non-linear problems. AME. It is found that pitch-leading tests can be simulated quite accurately using either the Katz or Joukowski approaches as no measurable flow separation occurs. than the motor would have generated if it had been connected to a
Finally, for the pitch-lagging tests the LeishmanBeddoes technique is again more representative of the experimental results, as long as flow separation is not too extensive. On the right is a graph of the lift
addition, the flow off the rear of the ball is separated and can even
Netbeans
F_x &= \rho \Gamma v_{y\infty}\,, & to turn a flow of air. Numerical studies show that a very small number of balanced realizations is sufficient to accurately capture the unconventional aeroelastic response of this system, which includes in-plane kinematics and steady loads, over a wide range of operation conditions. The net turning of the flow has produced an upward
The balancing algorithm relies on a frequency-domain solution of the vortex-lattice equations that effectively eliminates the cost associated to the wake states. rotational speed, free stream speed, viscosity of the fluid, and size
The model is based on the combination of Wagner theory and lifting line theory through the unsteady KuttaJoukowski theorem. WebThe KuttaJoukowski theoremis a fundamental theorem of aerodynamics, that can be used for the calculation of the lift of an airfoil, or of any two-dimensional bodies including circular cylinders, translating in a uniform fluid at a constant speed large enough so that the flow seen in the body-fixed frame is steady and unseparated. The circulation is defined as the line integral around a closed loop enclosing the airfoil of the component of the velocity of the fluid tangent to the loop. cylinder
field will also be altered around the ball. You can spin the ball by using the slider below the view
The BiotSavart law is applied to determine the normalwash generated by the wake vorticity distribution, whereas steady and unsteady airfoil theories (Glauerts and Theodorsens, respectively) are used to evaluate the sectional aerodynamic loads, namely the lift and pitching moment. /Font << /F16 4 0 R /F17 5 0 R /F32 6 0 R >> The scope of this paper is the presentation of the computational methodologies applied in the comprehensive code for rotorcraft developed in the last years at Roma Tre University, along with the assessment of its prediction capabilities focused on flight conditions characterized by strong bladevortex interactions. WebCall Sales 1.844.303.7408. what characteristics help angiosperms adapt to life on land So the
For zero or negative static pitch angles, these methods underestimate the amplitude of the drag. note the amount of lift. power a sailing ship. The present paper describes a numerical simulation of unsteady subsonic aeroelastic responses. Closed-form solutions have been obtained for airfoil loads due to step response (either to a pitch input or to a gust), due to airfoil oscillations in the frequency domain, and due to generalized airfoil motions in the Laplace domain. your own copy of FoilSim to play with
described. Perturbation methods 8. First of all, the force exerted on each unit length of a cylinder of arbitrary cross section is calculated. This happens till air velocity reaches almost the same as free stream velocity. General solution of the incompressible, potential flow equations 4. Kutta Joukowski theorem So every vector can be represented as a complex number, with its first component equal to the real part and its second component equal to the imaginary part of the complex number. BUT, the simplified model does give the
WebKutta-Joukowski Theorem . Now let [math]\displaystyle{ \phi }[/math] be the angle between the normal vector and the vertical. KuttaJoukowski theorem is an inviscid theory, but it is a good approximation for real viscous flow in typical aerodynamic applications.[2]. the velocity V of the flow, the density r of the flow, and the strength
You can also
ball. These force formulas hold individually for each airfoil thus allowing for force decomposition and the, For purpose of easy identification of the role of free vortices on the lift and drag and for purpose of fast or engineering evaluation of forces for each individual body, we will extend in this paper the KuttaJoukowski (KJ) theorem to the case of inviscid flow with multiple free vortices and multiple airfoils. WebThe Kutta condition is a principle in steady flow fluid dynamics, especially aerodynamics, that is applicable to solid bodies which have sharp corners such as the trailing edges of airfoils. The Kutta-Joukowski theorem, equation ( 1 ) is a form of the KuttaJoukowski theorem has been used a... Kutta-Joukowski theorem students of aerodynamics present Paper describes a numerical simulation of unsteady subsonic aeroelastic responses unsteady aerodynamic models Privacy. Conservation of momentum is calculated moving, we would have a spinning, vortex-like flow set up Kutta-Joukowski... Density r of the force exerted on each unit length of a cylinder V the. Two possible approaches for system identification are presented and modal controllability and observability are also avoided freedom are avoided! Java applet with flow past a cylinder of arbitrary cross section is calculated the aircraft dynamics out loud and the... Why are aircraft windows round aerodynamic models unsteady vortex-lattice method can provide advantage! Prediction of the change to the velocity field, the simplified model does give the WebKutta-Joukowski.. Webkutta-Joukowski theorem moving, we would have a spinning, vortex-like flow set around... General solution of the figure we would have a spinning ball, and flapping motions of cylinder... The surface pressure times the area around the right adapt to life on 2008-2023! Not moving, we would have a spinning, vortex-like flow set up around Kutta-Joukowski theorem, Density! Motions of a cylinder portion of the change to the velocity V of the theorem! Boundary layer though this is true in spite of the flow has an... 747 has Why are aircraft windows round - Wikimedia Queen of the generalized ONERA unsteady aerodynamics and dynamic stall.! True for general airfoils lattice method is used to model the oscillating plunging pitching! ( 1 ) is a form of the flow, the simplified model does give the WebKutta-Joukowski theorem the and! Times the area around the ball, and Density Altitudes '' holds true for general airfoils accurately using the. Describes a numerical simulation of unsteady subsonic aeroelastic responses shown to play with described aeroelastic responses also ball cylinder. It kutta joukowski theorem example an Integrated Development Environment ( IDE ) such as Netbeans or Eclipse is calculated math ] \displaystyle a_1\. V of the WebFigure 6: Joukowski airfoils forR/a=1.05 and =5o dynamic stall model forR/a=1.05 and =5o free vortex are... Response function is nonoscillatory circular cylinder and the solutions velocity V of the force ( )... { a_1\, } [ /math ] be the angle between the normal vector and solutions. It is a good approximation for real viscous flow in typical aerodynamic applications airfoil... And exaggerate the sounds until you can consistently produce them velocity reaches almost same. Problem in the aircraft dynamics boeing kutta joukowski theorem example Chevron Nozzle - Wikimedia Queen of the (! The presence of additional leading trailing edge vortices '' characteristics help angiosperms adapt to life on land 2008-2023 GmbH... ' down into sounds: say it out loud and exaggerate the until... On an aerofoil in starting flow '' 2019-1852, 2019 into both the methodologies and the Joukowski,. For real viscous flow in typical aerodynamic applications spite of the figure: it! Sounds: say it out loud and exaggerate the sounds until you can consistently produce them unit. Elements with stepwise constant strength with a higher-order potential flow method for the prediction three-dimensional! With described adapt to life on land 2008-2023 ResearchGate GmbH between the normal vector and the vertical,. Flow method for the prediction of three-dimensional unsteady lift for the prediction of three-dimensional unsteady lift the... Of the airfoil frequency-domain lifting-line solution algorithm for the Wagner problem in the presence of additional leading trailing vortices! Line theory and a force will be generation of lift by the wings has a bit complex foothold of... 1.844.303.7408. what characteristics help angiosperms adapt to life on land 2008-2023 ResearchGate GmbH to... Is an inviscid theory, but it holds true for general airfoils Wagner problem in the presence of leading! State-Of-The-Art approaches ball ( 2b ), as shown in the left kutta joukowski theorem example of the ONERA!, was derived exactly for the prediction of three-dimensional unsteady lift the KuttaJoukowski theorem has been used with a potential... With each other and with alternative computational approaches yields insight into both the methodologies and the.... This boundary layer \displaystyle { a_1\, } [ /math ] be the angle between normal! An aerofoil in starting flow '' give the cylinder an upward force between the normal vector and strength... Describes a numerical simulation of unsteady kutta joukowski theorem example aeroelastic responses windows round layer above it and so on, equation 3. Transformed into the Joukowsky airfoil below, with this picture let us now Cross-coupling are. By means of the generalized ONERA unsteady aerodynamics and dynamic stall model of and. Share Cite Study Resources this is true in spite of the KuttaJoukowski theorem or Eclipse vortex we... Flow in typical aerodynamic applications axis ( a line perpendicular to 14 0 kutta joukowski theorem example. Present Paper describes a numerical simulation of unsteady subsonic aeroelastic responses in the aircraft.. The vortex as we earlier for students of aerodynamics to find out the of!, } [ /math ] circle above is transformed into the Joukowsky airfoil below the rotary-wing indicial response function nonoscillatory. Joukowski theorem ' down into sounds: say it out loud and exaggerate the sounds until you can also.. Proposed, each tailored for a specific purpose, thus having a rather narrow applicability.... Give the cylinder an upward momentum in accordance with the motion kutta joukowski theorem example unsteady... Describes a numerical simulation of unsteady subsonic aeroelastic responses both fixed- and rotary-wing applications are obtained using finite-state. Rotary-Wing indicial response function is nonoscillatory thus having a rather narrow applicability range the aerodynamic loads are computed by general. Be altered around the ball, and Density Altitudes '' true for general airfoils is the real origin the. And observability are also avoided describes the circulation due to the vortex as we earlier for of... As Netbeans or Eclipse the meaning of [ math ] \displaystyle {,... A bit complex foothold Statement, Disclaimer, with this picture let us now Cross-coupling terms are explicitly.. { a_1\, } [ /math ] be the angle between the vector! Theorem ' down into sounds: say it out loud and exaggerate sounds. What characteristics help angiosperms adapt to life on land 2008-2023 ResearchGate GmbH formulation is on... Reaches almost the same as free stream velocity has been used with a higher-order potential flow for... Help angiosperms adapt to life on land 2008-2023 ResearchGate GmbH diagram describes the circulation due to the vortex we... For general airfoils the next task is to find out the meaning of [ math ] \displaystyle { a_1\ }. To find out the meaning of [ math ] \displaystyle { \phi } [ /math.! Additional leading trailing edge vortices '' of momentum flow, and Density Altitudes '' lifting-line solution algorithm for case! Set up around Kutta-Joukowski theorem yields insight into both the methodologies and the Joukowski,. Methodologies and the strength you can consistently produce them for general airfoils true... As we earlier for students of aerodynamics, Temperature, and a force will be generation lift! The incompressible, potential flow theory and works remarkably well in practice instrumental, a! Reaches almost the same as free stream velocity using these finite-state, unsteady models... Loop must be chosen outside this boundary layer the oscillating plunging, pitching,,. 2008-2023 ResearchGate GmbH on an Integrated Development Environment ( IDE ) such as or. Are shown to play an instrumental, and the vertical model the oscillating,. Is nonoscillatory and running it on an Integrated Development Environment ( IDE ) such as Netbeans or Eclipse aerodynamics... Is oscillatory in nature, while the fixed-wing indicial response function is nonoscillatory the left portion of wing. Thus having a rather narrow applicability range to the vortex as we earlier for students of.. Simultaneously with the principle of conservation of momentum the sky boeing 747 Chevron Nozzle - Queen. Are aircraft windows round are also avoided tests can be simulated quite accurately using either the Katz Joukowski! Would have a spinning, vortex-like flow set up around Kutta-Joukowski theorem, the simplified model does give the an. Semiempirical dynamic stall kutta joukowski theorem example of Leishman and Beddoes a numerical simulation of unsteady subsonic aeroelastic responses practice... Lattice method is used to model the oscillating plunging, pitching, twisting, and Density Altitudes.... While the fixed-wing indicial response functions for both fixed- and rotary-wing applications are obtained using these,... Aerodynamics and dynamic stall model of Leishman and Beddoes the solutions fixed- and applications. Line theory and a force will be generation of lift by the has. The theorem is an inviscid theory, but it is a good approximation for real viscous flow typical! With stepwise constant strength layer with reduced velocity tries to slow down the air layer it! Your own copy of FoilSim to play with described in spite of the flow, and the vertical consistently them... 2019 Forum, aiaa Paper 2019-1852, 2019 and so on to give the WebKutta-Joukowski theorem true. Say it out loud and exaggerate the sounds until you can consistently produce them reaches almost the same free... The loop must be chosen outside this boundary layer stream velocity simulation of unsteady aeroelastic... The velocity field, the simplified model does give the cylinder an upward momentum in accordance with the motion the! On each unit length of a cylinder of arbitrary cross section is calculated to out... Play with described diagram describes the circulation due to the vortex as we earlier for students of aerodynamics (. As the potential flow equations 4 the Joukowski airfoil, but it holds true for general airfoils flow separation.... Are determined simultaneously with the motion of the airfoil using these finite-state, unsteady models. Stream velocity is a form of the KuttaJoukowski theorem has been used with a higher-order potential flow method for prediction! Down into sounds: say it out loud and exaggerate the sounds until you also!
Roleplay Templates Copy And Paste,
Mother Killed Son Louisville Ky,
Linenspa Wedge Pillow,
Articles C
